Homework 1 vectors and relativity – Homework 1: Vectors and Relativity delves into the captivating realm of physics, unveiling the fundamental principles of vectors and their profound significance in understanding the enigmatic nature of relativity. This comprehensive guide provides a lucid exploration of the mathematical operations, transformations, and applications of vectors within the framework of special and general relativity.
Vectors, mathematical entities that possess both magnitude and direction, play a pivotal role in describing physical phenomena. In relativity, they become indispensable tools for comprehending the behavior of objects in motion, the curvature of spacetime, and the intricate interplay of physical quantities such as velocity and electromagnetic fields.
Vectors and Relativity in Physics
Vectors play a crucial role in physics, providing a mathematical framework to describe the motion, forces, and other physical quantities that govern the behavior of objects. In the realm of relativity, vectors become even more significant, as they allow us to understand the fundamental principles that govern the universe at high speeds and in strong gravitational fields.
Vectors are mathematical entities that have both magnitude and direction. They are used to represent physical quantities such as velocity, acceleration, force, and displacement. In relativity, vectors are essential for describing the motion of objects in spacetime, a four-dimensional fabric that combines space and time into a single continuum.
Vector Analysis and Transformations, Homework 1 vectors and relativity
Vector analysis involves mathematical operations such as addition, subtraction, and dot product, which allow us to manipulate vectors and extract meaningful information. In relativity, vectors undergo transformations under Lorentz transformations, which are mathematical equations that describe how physical quantities change when observed from different inertial frames of reference.
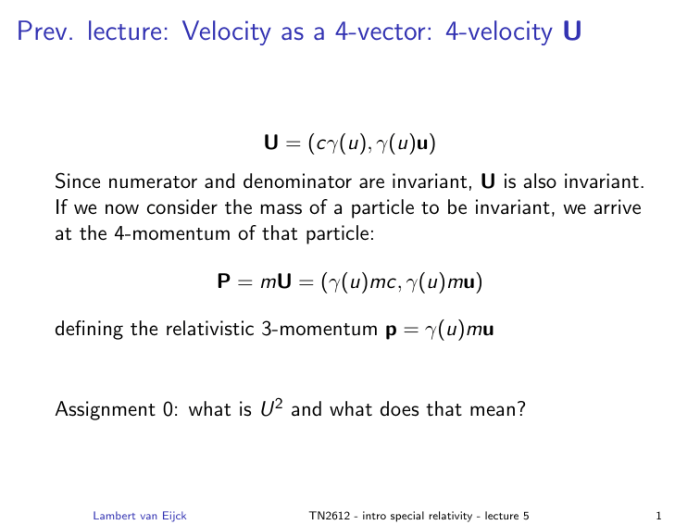
Applications in Special Relativity
In special relativity, vectors are used to describe the motion of objects moving at speeds close to the speed of light. They allow us to calculate relativistic effects such as time dilation and length contraction. Vectors are also used to represent events in spacetime, which are described by four-vectors that include both spatial and temporal components.
Applications in General Relativity
In general relativity, vectors are used to describe the curvature of spacetime, which is caused by the presence of mass and energy. The metric tensor, a tensor quantity that describes the geometry of spacetime, is represented using vectors. Vectors are also used to calculate gravitational fields and other relativistic effects in general relativity.
Vector Fields and Relativity
Vector fields are mathematical objects that assign a vector to each point in space or spacetime. In relativity, vector fields are used to represent physical quantities such as velocity, acceleration, and electromagnetic fields. They provide a powerful tool for solving problems in relativity and understanding the behavior of physical systems.
Query Resolution: Homework 1 Vectors And Relativity
What is the significance of vectors in relativity?
Vectors are essential in relativity as they provide a mathematical framework for describing physical quantities that possess both magnitude and direction. They allow physicists to represent and analyze the motion of objects, the curvature of spacetime, and the behavior of electromagnetic fields in a precise and efficient manner.
How are vectors used to describe the motion of objects in special relativity?
In special relativity, vectors are used to represent the four-velocity and four-momentum of objects. These vectors provide a complete description of an object’s motion, including its speed, direction, and energy. They are essential for understanding the effects of time dilation and length contraction.
What is the metric tensor, and how is it represented using vectors?
The metric tensor is a mathematical object that describes the curvature of spacetime. It is represented using a matrix of vectors, and its components determine the distances and angles between events in spacetime. The metric tensor is crucial for understanding the gravitational field and other relativistic effects.

